Imagine you are a second grade teacher and you have given this problem to your students as a task to start the day.

‍and in response, here is a sampling of your students’ responses:
‍

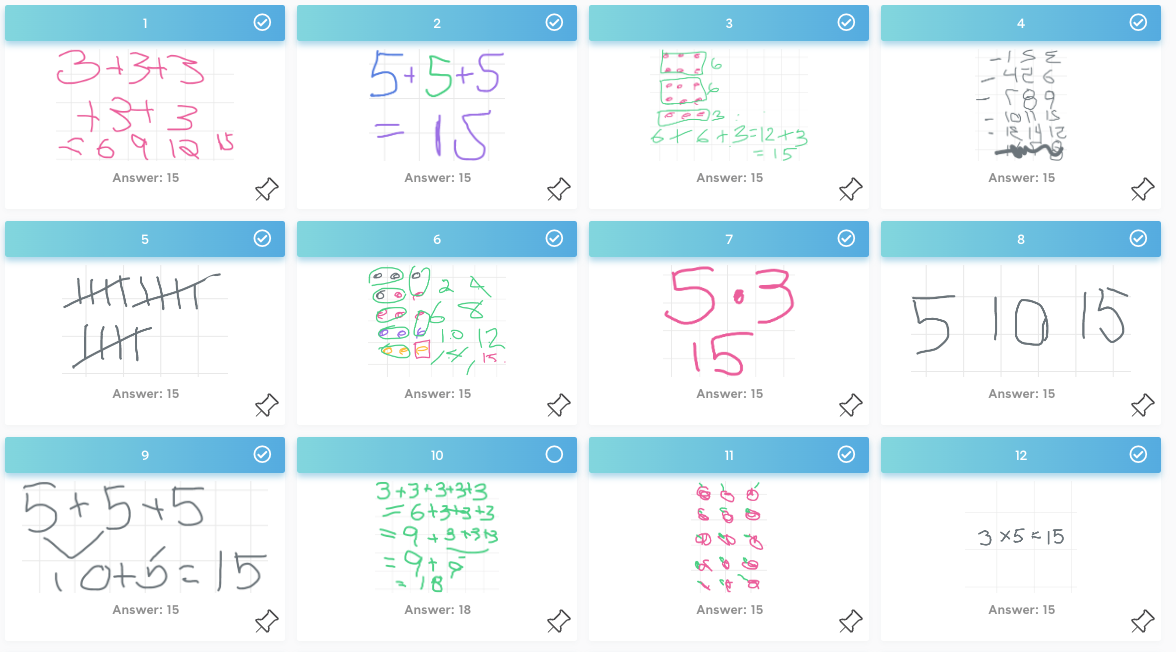
The question I am pondering is, what next? How will I use my students’ own work to build on our collective understanding of mathematics? What student work will I pin and show the class? What questions will I ask my students as we examine their work more closely.

‍It all depends on my goals for the lesson!

‍This problem is aligned to the second grade common Common Core Standards 2.OA 3 and 2.OA 4 Counting objects in arrays and equal groups. My goal for the unit might be having students work with equal groups of objects to gain foundations for multiplication. As in most classrooms, I have students with a range of understanding, which I can see in their work. 

Almost all of the students got the correct answer of 15. First, let’s think about student #10, who incorrectly answered 18. Looking at this student’s work closely - how on track with the learning goal are they? Am I worried? or, maybe do I hope this student at some point in the conversion will say, “That was me, and I see what I did! Can I revise my thinking?” 

Students #4, #5 and #11 appear to be counting by 1’s. Whose work in the class would we want to show them first and make connections with to support their learning? I would argue that we would not want to start by showing the work of students #7 and #12, since that won’t give all the students access to the thinking. 

Resources such as Graham Fletcher’s progression videos can help us make informed decisions about which student work to discuss in class and in what order. From this picture of his Multiplication Progression, I would want to start the discussion talking about repeated addition and then groups of 3 and/or 5. I would want to make the link to skip counting, and possibly explore the work of student #3 who has 2 and a half groups of 6.

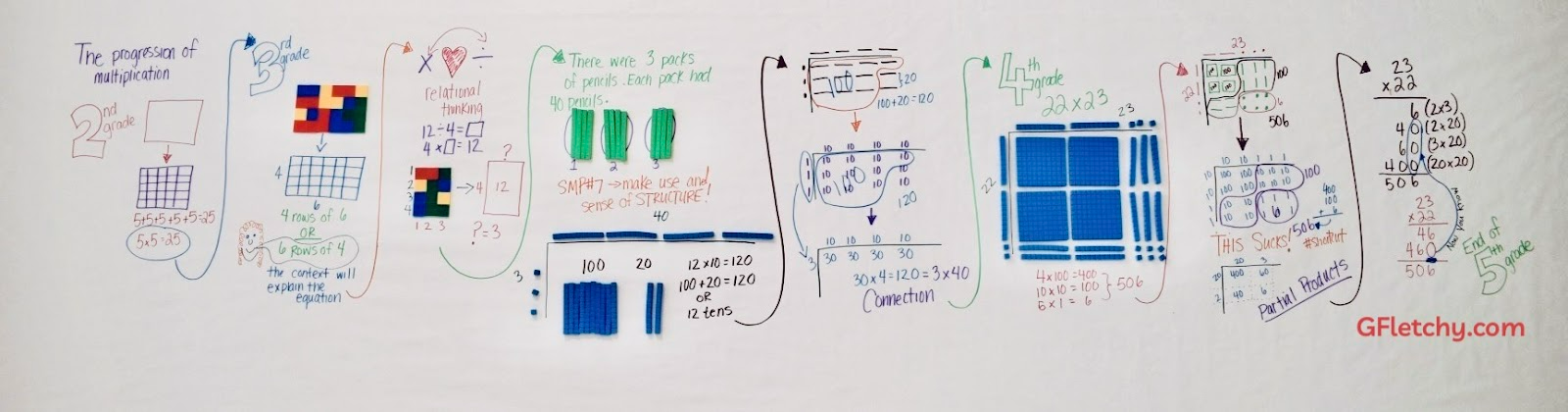
Another important thing to consider about my goals for the lesson is which of the Standards for Mathematical Practice (SMPs) am I addressing? As well as which SMPs “pop” out in the moment. I can’t help but think I would want to support SMP 7: Look for and make use of structure in a conversation based on the work of students #1 and #3, exploring how 3 + 3 + 3 + 3 + 3 is the same and/or different from 5 + 5 + 5. 

And last, but not least are my Social Emotional Goals for my students. As I look across my students’ work with their names showing, I wonder if there are any students who have not had an opportunity to shine lately. Are there students who need an opportunity to have their work highlighted and seen? 

The work of teaching is complex, and we teachers are reported to make about 1500 decisions a day! Having our goals with regard to the content standards and the Standards for Mathematical Practice, at both the unit and individual lesson level can help support us in that decision making process “at speed.” 

Best,
Leslie
leslie@magmamath.com